2/4 as a Decimal – A Comprehensive Guide

Have you ever wondered what the decimal equivalent of 2/4 is? While many might instinctively know the answer, others may find this simple fraction a bit confusing. As a lover of numbers and a passionate advocate for making math accessible to everyone, I’ve always found it fascinating how fractions can be transformed into decimals, and vice versa. This article will provide a clear and simple explanation of how to convert 2/4 to a decimal, accompanied by insightful examples and explanations that make the process easy to understand.

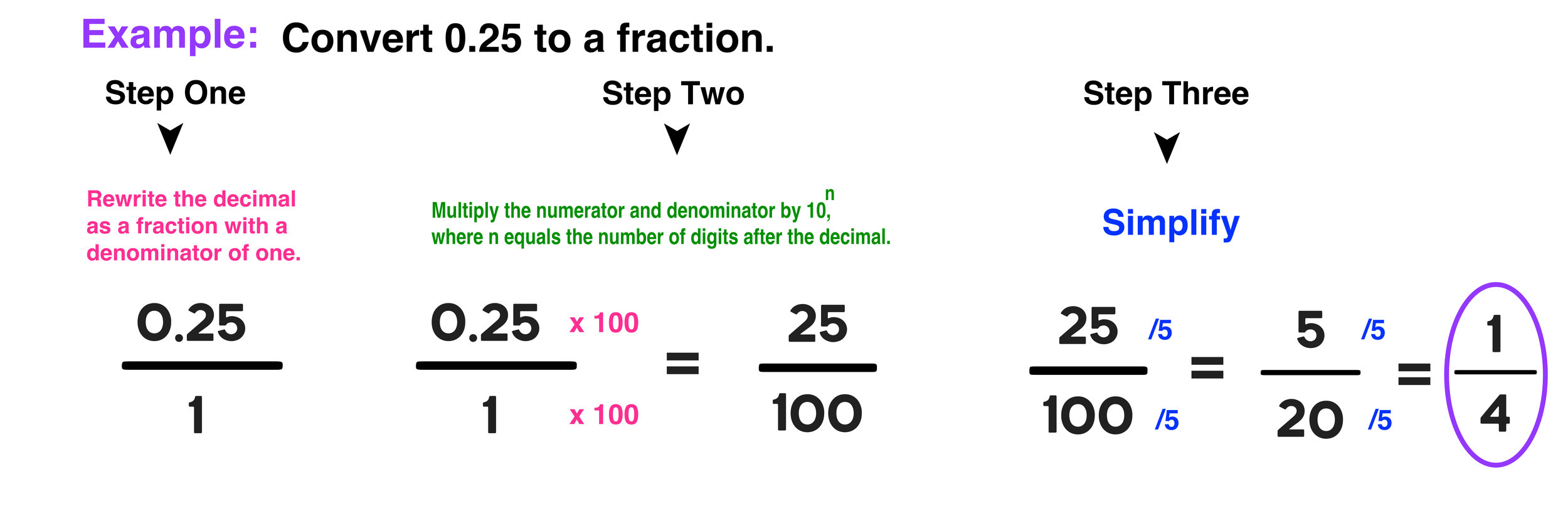
Image: calculator.oneplus.digital

Today, we will dive into the world of fractions and decimals, exploring the connection between these integral mathematical concepts. We’ll begin with a basic understanding of fractions and decimals and then embark on a journey to understand how to convert fractions like 2/4 into their decimal equivalents. I’ll introduce you to different methods for converting fractions to decimals, emphasizing clarity and ease of comprehension, making this conversion process a breeze for everyone!

Understanding Fractions and Decimals

Fractions and decimals represent different ways of expressing parts of a whole. A fraction consists of two parts: a numerator (the top number) and a denominator (the bottom number). The numerator shows how many parts of the whole you have, while the denominator indicates the total number of parts the whole is divided into.

Decimals, on the other hand, are based on the place value system. Each digit in a decimal represents a power of ten. The decimal point separates the whole number part from the fractional part. For instance, in the decimal 0.5, the 5 represents five tenths, or 5/10.

Converting 2/4 into a Decimal

There are two primary methods to convert 2/4 into a decimal:

Method 1: Division

The simplest method is to divide the numerator (2) by the denominator (4). This method is applicable to all fractions:

2 ÷ 4 = 0.5

Therefore, 2/4 is equivalent to 0.5 as a decimal.

Image: www.tes.com

Method 2: Simplifying the Fraction

Another approach involves simplifying the fraction by finding the greatest common factor (GCD) of the numerator and denominator. In this case, the GCD of 2 and 4 is 2. Dividing both the numerator and denominator by 2, we get:

2 ÷ 2 / 4 ÷ 2 = 1/2

Now, knowing that 1/2 represents half, we can easily convert it to its decimal equivalent: 0.5.

Why Understanding Decimals is Important

Understanding decimals is crucial for many daily life scenarios, such as:

• Financial calculations: Decimals are used in monetary transactions, representing cents or fractions of a dollar.
• Measurement: Units of measurement like centimeters and millimeters use decimals.
• Scientific calculations: Decimals are essential in expressing precise values and measurements in various fields like physics, chemistry, and engineering.
• Data analysis: Decimals often represent data points and statistics in graphs and charts.

Tips for Converting Fractions to Decimals

Here are a few tips to make converting fractions to decimals easier:

• Simplify the fraction first: Simplifying a fraction before division can make the calculation easier.
• Use a calculator: For complex fractions, a calculator can be a useful tool for quick conversions.
• Practice: The more you practice, the more comfortable you will become with converting fractions to decimals.

Converting fractions to decimals can sometimes feel daunting, especially when dealing with more complex fractions. Remember, take it one step at a time by simplifying the fraction if possible, using the division method, and utilizing a calculator if necessary. With consistent practice, converting fractions to decimals will become second nature to you.

FAQs about Fractions and Decimals

Q: What is the difference between a fraction and a decimal?

A: **Fractions** represent parts of a whole using a numerator and a denominator, while **decimals** represent parts of a whole using a decimal point and digits to the right of it. Both systems represent portions of a whole, but decimals are often preferred for calculations and representing precise values.

Q: How do I convert a mixed number (like 1 1/2) to a decimal?

A: To convert a mixed number to a decimal, first convert the fractional part as explained earlier. Then add the whole number part to the resulting decimal. So, for 1 1/2, converting 1/2 to decimal (0.5) and adding it to the whole number part (1) gives you 1.5.

Q: Are there any fractions that cannot be converted to decimals?

A: All fractions can be converted into decimals, but some fractions result in repeating decimals. For example, 1/3 is a fraction that results in 0.333333…, where the ‘3’ repeats infinitely.

2 4 As A Decimal

https://youtube.com/watch?v=A3slshaw2s4

Conclusion

Converting 2/4 to a decimal, which equals 0.5, is a straightforward process that can be achieved through division or simplifying the fraction. As we’ve seen, understanding decimals is critical for everyday life, and mastering the conversion process from fractions to decimals empowers you to navigate various mathematical applications with ease.

Are you ready to explore other fractions and decimal conversions? Share your thoughts and questions in the comments below!