Have you ever wondered what the building blocks of a number are? What smaller numbers combine to create a larger one? This is the essence of exploring factors, those numbers that divide evenly into a given number. In this article, we delve into the fascinating world of the factors of 200, uncovering their secrets and exploring their significance.
Image: everettnewsfinley.blogspot.com
Understanding factors is essential in various mathematical concepts, from simplifying fractions to solving equations. The ability to determine a number’s factors allows us to analyze its properties, identify patterns, and gain a deeper understanding of its numerical relationships. Let’s embark on a journey to unravel the factors of 200, revealing their hidden connections and practical applications.
Defining Factors: The Building Blocks of Numbers
Before diving into the specific factors of 200, let’s establish a clear understanding of what a factor is. In simple terms, a factor of a number is any number that divides evenly into it, leaving no remainder. For instance, the factors of 12 are 1, 2, 3, 4, 6, and 12, because each of these numbers divides evenly into 12.
The concept of factors is fundamental in arithmetic and is related to other important mathematical concepts, such as divisibility, prime numbers, and least common multiples. By understanding factors, we can gain valuable insights into the structure and properties of numbers, paving the way for further exploration and discovery in the world of mathematics.
Finding the Factors of 200: A Step-by-Step Guide
Now, let’s focus on the prime factor factorization of the number 200. This involves breaking down 200 into its prime factor components, which are numbers that are only divisible by 1 and themselves. To find the prime factors of 200, we can use the following steps:
1. Prime Factorization
Start by dividing 200 by the smallest prime number, which is 2. 200 divided by 2 equals 100. Since 100 is also divisible by 2, we continue this process until we reach a prime number:
- 200 ÷ 2 = 100
- 100 ÷ 2 = 50
- 50 ÷ 2 = 25
- 25 ÷ 5 = 5
This gives us the prime factorization of 200 as 2 x 2 x 2 x 5 x 5, or 23 x 52. This indicates that 200 is composed of three factors of 2 and two factors of 5.
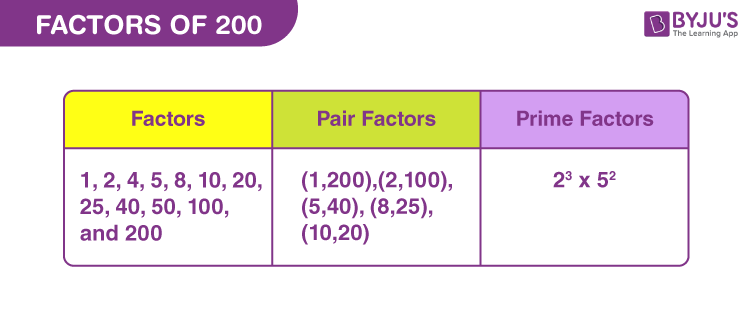
Image: byjus.com
2. Finding All the Factors
To determine all the factors of 200, we can utilize the following method:
1. **Consider each prime factor and add 1 to its exponent.** In our case, the exponents are 3 for 2 and 2 for 5. Adding 1 to each of them gives us 4 and 3, respectively.
2. **Multiply the results together.** 4 x 3 = 12. This tells us that 200 has a total of 12 factors.
3. **List all the factors.** To find the actual factors, we can systematically combine the prime factors using their exponents. Here’s how:
- Start with 20 x 50 = 1
- Move up the powers of 2: 21 x 50 = 2
- Continue with 22 x 50 = 4
- And 23 x 50 = 8
Now, move to the powers of 5:
- 20 x 51 = 5
- 21 x 51 = 10
- 22 x 51 = 20
- 23 x 51 = 40
Finally, use the highest power of 5:
- 20 x 52 = 25
- 21 x 52 = 50
- 22 x 52 = 100
- 23 x 52 = 200
Therefore, the factors of 200 are: 1, 2, 4, 5, 8, 10, 20, 25, 40, 50, 100, and 200.
Exploring the Significance of Factors
Understanding factors is crucial for various mathematical applications and real-life scenarios. Here are some examples:
1. Simplifying Fractions
Factors play a vital role in simplifying fractions. To simplify a fraction, we find the greatest common factor (GCF) of the numerator and denominator and divide both by this factor. For example, to simplify the fraction 200/400, we first find the GCF of 200 and 400, which is 200. Dividing both numerator and denominator by 200 gives us the simplified fraction 1/2.
2. Identifying Prime Numbers
A prime number is a number greater than 1 that is only divisible by 1 and itself. Understanding factors helps us identify prime numbers, as they have only two factors: 1 and themselves. For instance, the number 7 is prime because its only factors are 1 and 7.
3. Finding Least Common Multiples (LCM)
The least common multiple (LCM) of two numbers is the smallest number that is a multiple of both numbers. To find the LCM, we can use the prime factorizations of the numbers. We take the highest power of each prime factor that appears in either factorization. For example, to find the LCM of 200 and 150, we first find their prime factorizations: 200 = 23 x 52 and 150 = 2 x 3 x 52. The LCM is then 23 x 3 x 52 = 600.
4. Solving Equations
Factors are essential in solving equations, particularly those involving polynomials. Factoring a polynomial means expressing it as a product of simpler polynomials. This can help simplify equations and make them easier to solve. For example, the equation x2 – 4 can be factored as (x + 2)(x – 2). Setting each factor equal to zero gives us the solutions x = -2 and x = 2.
Real-World Applications of Factors
Beyond their mathematical significance, factors have practical applications in diverse fields:
1. Division and Sharing
In everyday life, we encounter factors when dividing objects or quantities evenly. For instance, if we have 200 cookies and need to divide them equally among 10 friends, each friend gets 20 cookies. This represents a factor of 20, as 200 is divisible by 20.
2. Measurement and Units
Factors are often used in measurements and conversions. For example, when converting between units like inches and feet, we rely on the factor of 12 (1 foot = 12 inches). This factor allows us to accurately translate between different units.
3. Computer Science and Algorithms
Factors are crucial in computer science and algorithms, where they play a role in developing efficient sorting and search algorithms. Understanding factors is essential for optimization and improving the performance of computer programs.
Factors Of 200
Conclusion
The journey of exploring factors has revealed their hidden secrets and practical significance. By understanding the concept of factors and how to find them, we gain valuable insights into the world of numbers and their relationships. From simplifying fractions to solving equations and making practical decisions in everyday life, factors provide a fundamental building block for various mathematical applications and real-world scenarios. As we continue to delve into the realm of mathematics, we discover the profound impact of understanding factors and their applications within complex systems and processes.



/GettyImages-173599369-58ad68f83df78c345b829dfc.jpg?w=740&resize=740,414&ssl=1)


