Unveiling the Secrets of 83 – A Deep Dive into Its Factors

Have you ever wondered about the building blocks of numbers? Numbers, much like complex structures, are composed of smaller units. These units, known as factors, are the integers that divide evenly into a larger number without leaving a remainder. Today, we embark on a fascinating exploration of the factors of 83, delving into its numerical properties and uncovering the intriguing relationships it holds within the realm of mathematics.

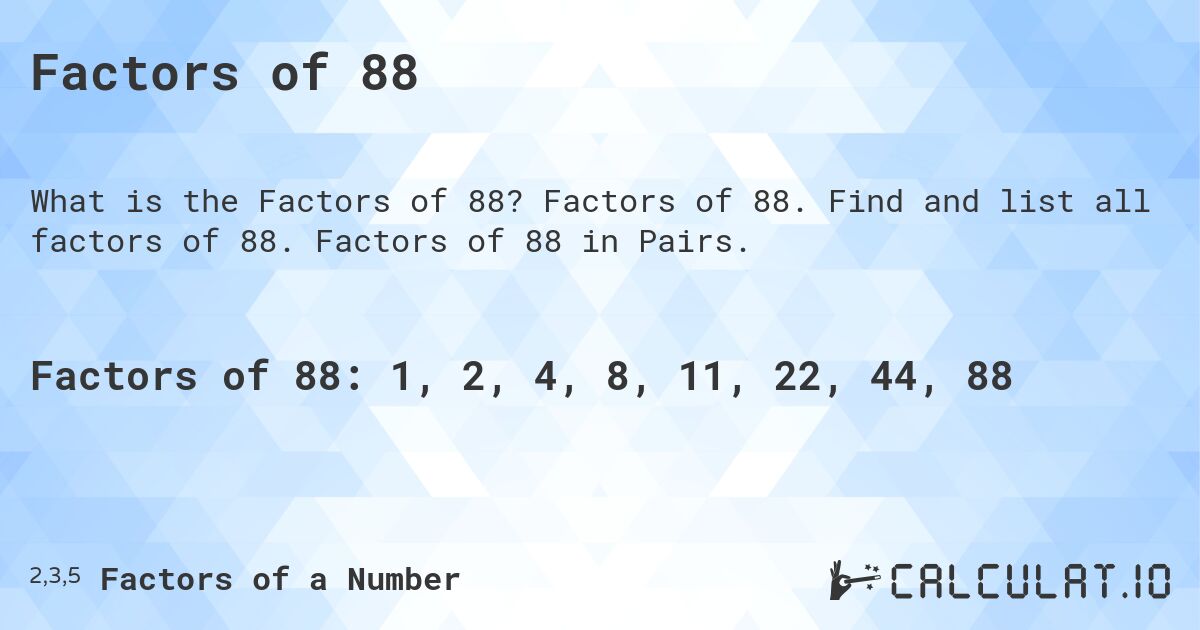
Image: calculat.io

Understanding factors plays a crucial role in various mathematical disciplines, from simple arithmetic to more advanced concepts like factorization and prime numbers. Whether you’re a student tackling your first algebra problem or a seasoned mathematician exploring the intricacies of number theory, grasping the concept of factors is essential. In this comprehensive journey, we will unravel the secrets of 83, elucidating its unique factorization and highlighting its significance within the broader landscape of mathematics.

Defining Factors: The Building Blocks of Numbers

Before embarking on our exploration of the factors of 83, let’s solidify our understanding of what constitutes a factor. In simpler terms, a factor is a number that divides evenly into another number, leaving no remainder. Consider the number 12. Its factors are 1, 2, 3, 4, 6, and 12, because each of these numbers divides into 12 without leaving a remainder.

Factors are the essential building blocks of numbers, much like bricks form a wall. They provide a fundamental way to understand the relationships between numbers and unravel their divisibility properties. The process of finding the factors of a number is known as factorization, which plays a critical role in various mathematical operations and problem-solving strategies.

Prime Numbers: The Unbreakable Building Blocks

Within the vast universe of numbers, prime numbers occupy a special place. These unique numbers are divisible only by 1 and themselves. Examples of prime numbers include 2, 3, 5, 7, 11, and 13. Prime numbers are often referred to as the ‘atomic’ elements of numbers, as they cannot be broken down further into smaller factors.

The fundamental theorem of arithmetic states that every integer greater than 1 can be uniquely expressed as a product of prime numbers. This theorem underscores the importance of prime numbers as the fundamental building blocks from which all other integers are constructed. Understanding prime numbers is crucial for deciphering the structure of numbers and unraveling their intricate relationships.

The Uniqueness of 83: A Prime Number’s Tale

Now, let’s turn our attention to our subject, the number 83. What makes 83 unique is its status as a prime number. This means that the only factors of 83 are 1 and 83 itself. Unlike other numbers that can be broken down into multiple factors, 83 stands alone as an indivisible element.

The fact that 83 is a prime number reveals a fundamental truth about its nature – it cannot be expressed as a product of two smaller integers. This characteristic sets 83 apart from composite numbers, which can be factored into smaller numbers. The primality of 83 underscores its unique position within the number system, highlighting its indivisible and foundational nature.

Image: findthefactors.com

Exploring the Concept of Factorization

Factorization, the process of finding the factors of a number, is a fundamental concept in mathematics. It’s employed in various branches of mathematics, including algebra, number theory, and cryptography. Factorization involves breaking down a number into its smaller components, which can be either prime numbers or other factors.

For instance, let’s consider the number 24. We can factorize 24 as follows:

• 24 = 2 x 12
• 12 = 2 x 6
• 6 = 2 x 3

By breaking down 24 into its prime factors, we have identified the building blocks of this composite number: 2 x 2 x 2 x 3. This factorization process reveals the fundamental structure of 24 and provides valuable insights into its divisibility properties.

Prime Factorization: The Foundation of Number Theory

Prime factorization plays a pivotal role in number theory, the branch of mathematics dedicated to studying the properties of integers. By factoring a number into its prime components, we gain deep insights into its divisibility, relationships with other numbers, and various mathematical properties. This process forms the backbone of understanding the intricate structure and relationships within the number system.

For instance, understanding the prime factorization of a number can help us determine its greatest common divisor (GCD) and least common multiple (LCM) with another number. These concepts are crucial in various mathematical operations, including simplifying fractions, finding the lowest common denominator, and solving algebraic equations.

Applications in Real World Scenarios

The concept of factors and factorization extends beyond the realm of abstract mathematics. It finds practical applications in various real-world scenarios:

• Cryptography: Prime numbers play a crucial role in modern cryptography, the science of secure communication. For instance, the RSA algorithm, widely used for secure communication over the internet, relies on the difficulty of factoring large numbers into their prime components.
• Computer Science: Factorization is utilized in various areas of computer science, including algorithms for data compression, code optimization, and network security.
• Engineering: Factors are used in engineering for calculations related to structural design, load distribution, and material strength.
• Everyday Life: We encounter factors in everyday life, such as when dividing a cake into equal slices, splitting a bill among friends, or organizing items into groups.

Factors Of 83

The Significance of Understanding Factors

In conclusion, understanding the concept of factors is essential for various mathematical applications and real-world scenarios. Factors provide a fundamental way to analyze numbers, revealing their structure, relationships, and divisibility properties. From basic arithmetic to advanced cryptography, the concept of factors permeates many aspects of mathematics and its applications.

While the number 83 may appear simple at first glance, its status as a prime number reveals a profound truth about its indivisible nature. By exploring the factors of numbers like 83, we delve deeper into the intricacies of mathematics and appreciate the elegance and interconnectedness of the number system.

We encourage you to explore further, delve into the world of prime numbers, and discover the fascinating relationships they hold within the realm of mathematics. Let the quest for understanding factors guide you on an intellectual journey filled with discoveries and enlightenment.