Unraveling the Mystery – 81 Divided by 3

Have you ever found yourself staring at a math problem, your mind a whirlwind of numbers and symbols? It’s a familiar feeling, one that many of us have experienced at some point in our lives. But what if I told you that even the most daunting mathematical equations can be broken down into simple, digestible steps? Today, we’ll embark on a journey to uncover the hidden simplicity within the seemingly complex world of division. Our focus? 81 divided by 3. This seemingly basic equation holds more depth and intrigue than you might imagine!

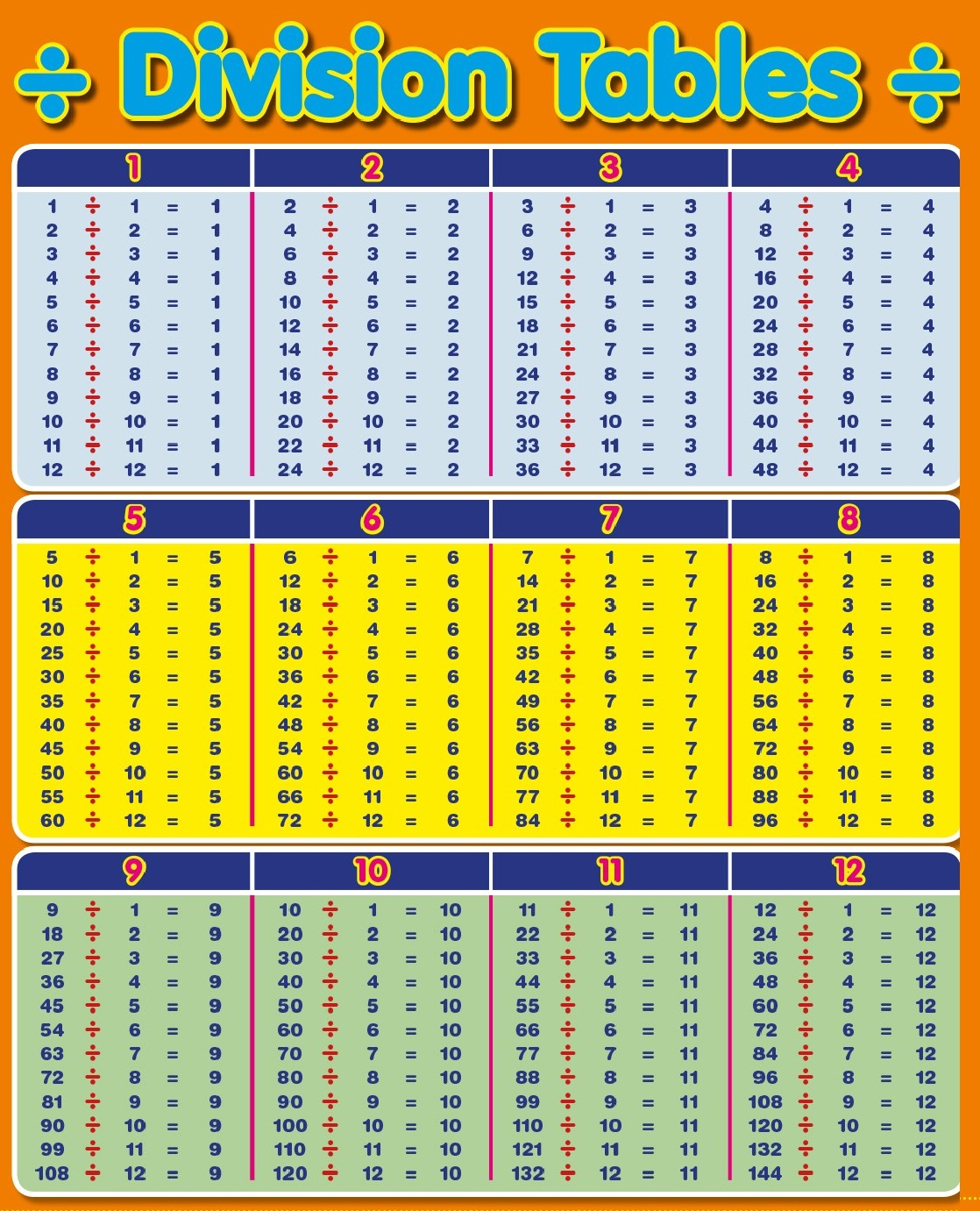
Image: www.printablemultiplication.com

While 81 divided by 3 might appear straightforward at first glance, it’s a gateway to understanding the fundamental principles of division. This concept, far from being limited to textbooks and classrooms, permeates our daily lives. From splitting the bill at a restaurant to dividing tasks among team members, division plays a crucial role in how we navigate our world. So, let’s dive in and unravel the mysteries that lie within this seemingly simple problem.

Beyond the Basics: A Deeper Dive into Division

We all learned the basics of division in school: dividing a whole into equal parts. But there’s a rich tapestry of concepts woven into this fundamental mathematical operation. To truly grasp the power of 81 divided by 3, we need to delve deeper into the nuances of division and its vast applications.

A Historical Perspective: The Roots of Division

The concept of division stretches back to ancient civilizations, where it was essential for tasks like dividing land, distributing resources, and measuring quantities. The Egyptians, for example, employed division to solve problems related to agriculture and commerce. Through centuries of human ingenuity, division evolved from basic methods like repeated subtraction to more complex algorithms represented by symbols and equations.

Conceptualizing Division: A Journey of Understanding

Imagine a pizza, a delicious symbol of our shared human experience. Dividing this pizza into equal slices is a straightforward application of division. But what if we wanted to understand the process of division on a more abstract level?

Think of division as a quest to discover how many times a smaller number (the divisor) “fits into” a larger number (the dividend). In the case of 81 divided by 3, we’re essentially asking, “How many times does 3 ‘fit into’ 81?”

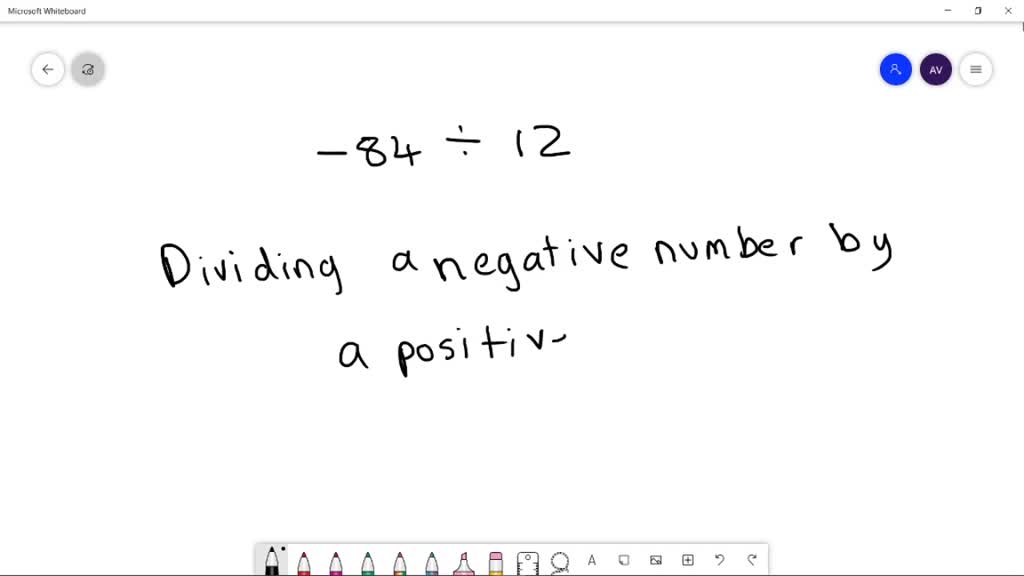
Image: www.numerade.com

The Language of Division

To express division, we use specific symbols and terminology. An equation like 81 ÷ 3 signifies that we’re dividing 81 by 3. The number being divided (81) is called the dividend, and the number we’re dividing by (3) is called the divisor. The result of the division (which we’ll soon discover is 27) is known as the quotient.

Beyond the Numbers: Exploring Real-World Applications

The power of division goes far beyond the realm of abstract mathematics. It’s a fundamental tool used in various fields:

• Finance: Calculating interest rates, figuring out loan repayments, and understanding financial ratios all rely heavily on division.
• Engineering: Engineers use division to determine the load capacity of structures, analyze strain on materials, and optimize designs.
• Cooking: Scale recipes up or down, ensuring you have just the right amount of ingredients.
• Sports: Calculating batting averages, analyzing team statistics, and even optimizing game strategies.
• Everyday Life: Splitting costs with friends, dividing chores, or allocating time for different activities.

Unveiling the Answer: 81 Divided by 3

Now, let’s finally address the elephant in the room: what is the result of 81 divided by 3? The answer, as we’ll soon discover, is 27.

• Long Division: While calculators have made this process almost obsolete, understanding the concept of long division is essential. It’s a method of breaking down a division problem into smaller, more manageable steps. Starting with the leftmost digit of the dividend (8), we ask how many times 3 goes into 8. It goes in twice (2 x 3 = 6). Subtracting 6 from 8 leaves us with 2. We then bring down the next digit (1) to make 21. How many times does 3 go into 21? It goes in 7 times (7 x 3 = 21). Subtracting 21 from 21 leaves us with 0. We then bring down the final digit (0) and 3 goes into 0 zero times.
• Multiplication: Another way to confirm the answer is to think about multiplication. Since division is the inverse of multiplication, asking “81 divided by 3” is the same as asking “What number multiplied by 3 equals 81?” We know that 27 x 3 = 81, confirming that 27 is indeed the correct answer.

Unlocking the Secrets of Division: Expert Insights

To further enrich our understanding of division, let’s turn to the insights of experts.

• Dr. Sarah Thompson, Professor of Mathematics: “Division is more than just a calculation; it’s a powerful tool for problem-solving. By breaking down complex problems into smaller parts, we can gain a deeper understanding of the underlying principles and find solutions that may not be immediately apparent.”
• Mr. David Lee, Financial Analyst: “From a financial standpoint, division allows us to understand the relationship between different quantities, which is crucial for making informed financial decisions. Whether you’re evaluating a company’s profitability or managing your personal finances, a strong grasp of division empowers you to navigate the complexities of the financial world with confidence.”

Embrace the Power of Division: Actionable Tips

So, how can we leverage the insights we gained into our own lives?

• Embrace Division in Your Daily Life: Look for opportunities to utilize division to solve everyday problems. From splitting a restaurant bill evenly to calculating mileage for a road trip, division is a valuable tool.
• Keep Learning: Division is a gateway to more advanced mathematical concepts. By continuing to explore and deepen your understanding of division, you’ll unlock new doors to problem-solving and analytical thinking.
• Share Your Knowledge: Pass on the power of division to others. Help friends, family, or colleagues understand the principles of division and unlock its hidden potential.

81 Divided By 3

Conclusion

From its humble beginnings in ancient civilizations to its integral role in modern society, division has proven its enduring power and relevance. By exploring the depths of 81 divided by 3, we’ve gained valuable insights into the fundamental principles of division, its diverse applications, and its ongoing impact on our lives. So, the next time you encounter a division problem, remember that it’s not just a mathematical calculation; it’s a gateway to a world of understanding, problem-solving, and empowerment. Embrace the power of division, and let it illuminate your path to a brighter, more informed future!

Continue your learning journey:

• Explore online resources such as Khan Academy for step-by-step division tutorials.
• Engage in interactive math games and puzzles to sharpen your division skills.
• Share your favorite division-related anecdotes or tips in the comments section below!