The Mysterious Swap – Unraveling the Secrets of dx/dy vs dy/dx

Ever found yourself staring at a calculus problem, wondering why on earth someone would swap the “d”s in a fraction like that? It’s a common question, especially for those just diving into the world of derivatives. But the question isn’t as simple as it might first appear. The difference between dx/dy and dy/dx goes far beyond a simple alphabetical switch; it’s a fundamental shift in perspective that dramatically alters how we perceive and work with functions.

Image: study.com

Imagine a map – a visual representation of different locations. Just like this map, we use equations to express relationships between variables. dx/dy and dy/dx are essentially like two different compasses on this map, offering distinct directions to navigate through the intricacies of these relationships. Understanding their differences is key to truly understanding the power and flexibility of calculus in describing change.

The Building Blocks: Understanding Derivatives

Before we embark on the journey of comparing dx/dy and dy/dx, let’s establish a solid foundation. At its core, calculus revolves around the concept of change. Derivatives are the tools that allow us to precisely measure and understand this change. They tell us how much one variable changes in relation to another.

Imagine a car driving down a highway. Its speed is a measure of how its position changes over time. We can think of position as our ‘y’ variable and time as our ‘x’ variable. The derivative dy/dx in this case represents the car’s instantaneous speed, telling us its velocity at any given moment.

The Flip Side: Unveiling the Power of dx/dy

While dy/dx is often our starting point in calculus, dx/dy offers a unique perspective. It’s essentially a change of perspective, allowing us to explore relationships from a different angle. Instead of asking “How does ‘y’ change with respect to ‘x’?” we now ask, “How does ‘x’ change with respect to ‘y’?”

Think of the car example again. Instead of focusing on its speed, dx/dy would reveal the time it takes the car to travel a specific distance. It answers the question, “How does time change as we shift along the path?”

The Power of Perspective: Applications Across Disciplines

The difference between dx/dy and dy/dx may seem subtle, but its implications are far-reaching, impacting diverse fields like economics, physics, biology, and engineering.

• Economics: In economics, dy/dx helps us understand the concept of marginal utility. By examining how the amount of satisfaction someone derives from a product changes with increasing consumption, we can determine the optimal consumption levels. dx/dy, on the other hand, helps us analyze the relationship between price and demand, revealing how changes in price influence the quantity of goods buyers are willing to purchase.
• Physics: In physics, dy/dx is crucial for understanding motion, like acceleration – the rate at which velocity changes. dx/dy can help us determine how the position of an object changes in response to its displacement.
• Biology: dy/dx is used to model population growth, allowing scientists to understand how population size changes over time. Alternatively, dx/dy can help us understand the relationship between the size of an organism and its lifespan, providing valuable insights into biological processes.

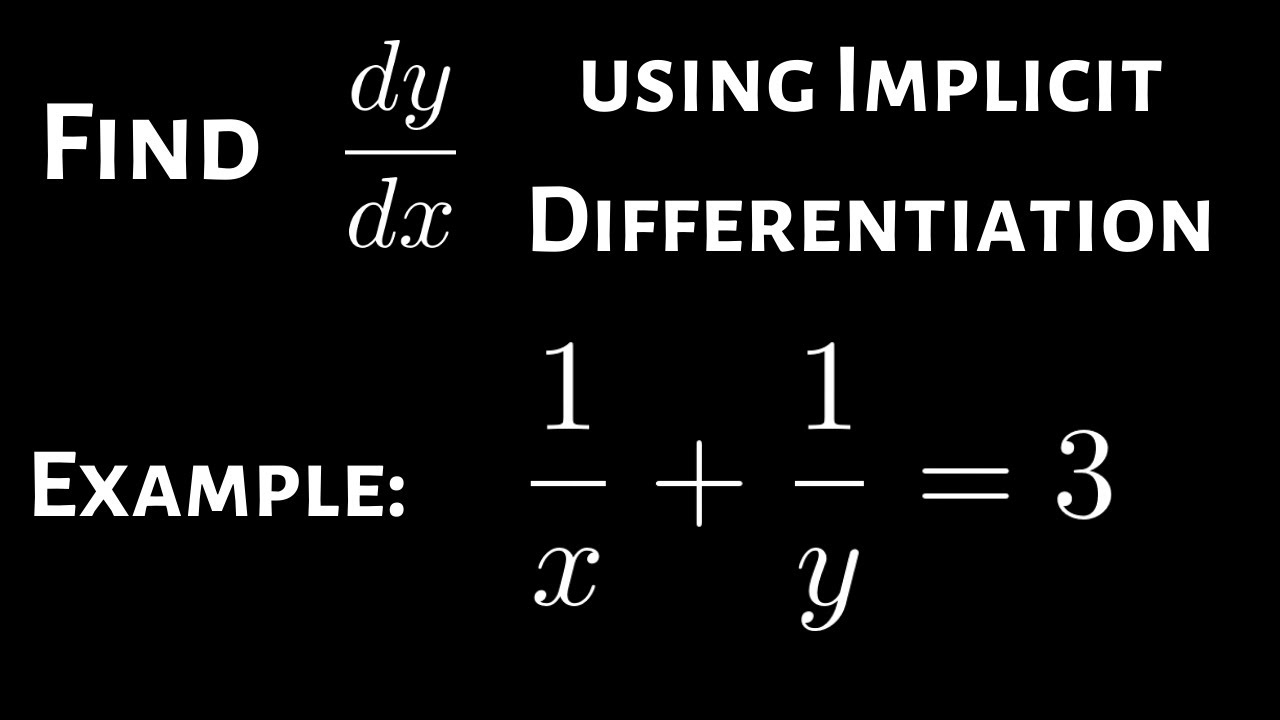
Image: www.youtube.com

Beyond the Basics: Advanced Concepts and Applications

The world of derivatives extends beyond simple single-variable functions. dx/dy and dy/dx find their place in more complex scenarios involving multiple variables and partial derivatives. These concepts are fundamental to understanding topics like:

• Optimization: Determining the maximum or minimum values of functions, finding the optimal solutions in various scenarios.
• Implicit Differentiation: Finding derivatives of equations that cannot be easily rearranged to explicitly represent one variable in terms of another, crucial in understanding the relationships between several interconnected variables.
• Curve Sketching: Understanding the behavior of functions by analyzing their slopes, concavity, and points of inflection.

Dx/Dy Vs Dy/Dx

Conclusion: Navigating the World of Derivatives

Understanding the difference between dx/dy and dy/dx is crucial for truly mastering calculus and its vast applications. Each perspective offers a unique lens through which we can analyze the dynamic world of change. By embracing the power of both, we unlock a wider understanding of how quantities interact, enabling us to solve complex problems and make insightful predictions in diverse fields.

So, the next time you encounter these seemingly simple fractions, remember that they represent more than just a swap of letters. They represent a change in perspective, a journey through different dimensions of understanding, helping us unlock the secrets hidden within the world of change and its fundamental nature.