Unraveling the Mystery of dx dy – A Journey into the Heart of Calculus

Have you ever stared at a mathematical equation and felt a wave of confusion wash over you? Imagine seeing “dx dy” scribbled on a whiteboard, or maybe even in a textbook, and feeling utterly lost. You’re not alone. This seemingly cryptic symbol has haunted countless students and even seasoned mathematicians. But fear not, dear reader, for today we embark on a journey to demystify this mathematical enigma. We will journey into the heart of calculus and unravel the captivating story of dx dy, revealing its true meaning and power.

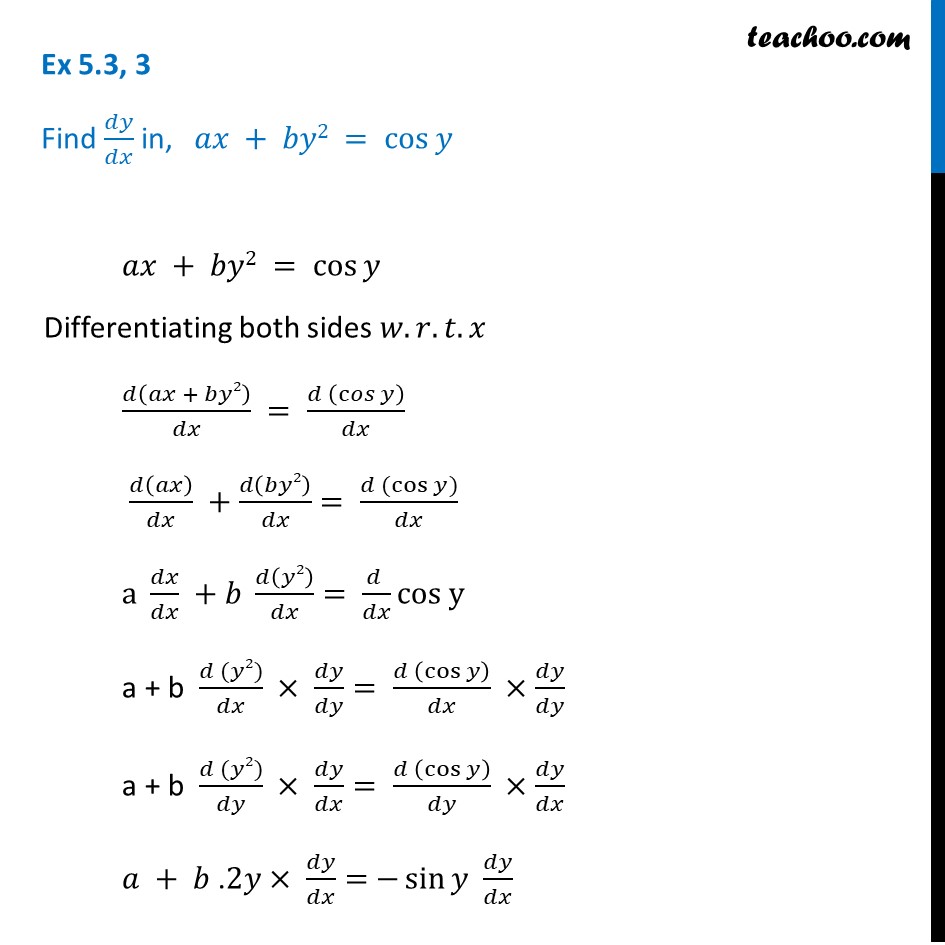
Image: www.teachoo.com

At its core, “dx dy” represents a fundamental concept in calculus, the language of change. It’s a key player in the world of derivatives and integrals, which allow us to measure rates of change and calculate areas and volumes. But what exactly do those tiny “dx” and “dy” represent? Imagine zooming in on a graph, focusing on a tiny sliver of the curve. These tiny symbols represent those incredibly small increments of change along the x and y axes. They are the building blocks of calculus, allowing us to understand how quantities evolve and interact. So, with a newfound understanding, let’s delve deeper into the fascinating world of dx dy and explore its significance.

A Glimpse into the Past: The Genesis of dx dy

The concept of “dx dy” finds its roots in the pioneering work of mathematicians like Isaac Newton and Gottfried Wilhelm Leibniz. These giants of the scientific revolution laid the groundwork for calculus, revolutionizing our understanding of the universe. They paved the way for the development of calculus, a powerful tool for understanding change and motion. Newton’s investigations into the motion of objects led to his development of the concept of a derivative, the instantaneous rate of change. Leibniz, independently, developed a similar concept, known as the differential, which is intimately linked to the “dx dy” we are exploring.

Imagine a moving object. Newton realized that its velocity, the rate of change of its position, could be determined by considering infinitesimally small changes in both time (“dt”) and distance (“ds”). This was the genesis of the derivative, expressed as ds/dt, the ratio of infinitesimal changes. Leibniz’s approach was similar, focusing on “infinitesimal differences” and representing these changes using “dx” and “dy”. These early mathematicians didn’t have the luxury of modern notation, but their insights laid the foundation for the calculus we know today. While dx dy is often used in a broader context to represent these infinitesimal changes, the notation embodies the spirit of their groundbreaking work.

The Power of Infinitesimal Change: Decoding dx dy

“dx dy” is a powerful symbol that unlocks a treasure trove of mathematical possibilities. It’s the key to understanding how functions change and interact. Picture a smooth, flowing curve on a graph. The “dx” represents a tiny step or change along the x-axis, while the “dy” represents the corresponding change on the y-axis. This small change on the y-axis, “dy,” is determined by the slope of the curve at that very point, which is where the derivative comes in. The derivative, represented as dy/dx, embodies the relationship between these infinitesimal changes. This simple ratio holds the key to understanding how the function changes, revealing its rate of change at any given point.

The Art of Change: Exploring Applications of dx dy

The applications of “dx dy” are vast and profound, touching almost every aspect of our world. From predicting the path of a rocket in space to modeling the spread of infectious diseases, this tiny mathematical symbol plays a pivotal role.

• Physics: In physics, dx dy is essential for describing motion, work, and energy. These tiny changes in distance and time are fundamental to understanding classical mechanics.
• Engineering: The concept of the derivative, encapsulated by dx dy, is essential in fields such as structural engineering, where determining rates of change in materials like steel is vital for designing safe bridges, skyscrapers, and other structures.
• Economics: dx dy helps us to understand how a change in one variable affects another variable, offering crucial insights into market dynamics and economic growth.
• Biology: In biological sciences, dx dy plays a role in modeling population growth, the spread of diseases, and the dynamics of ecosystems.

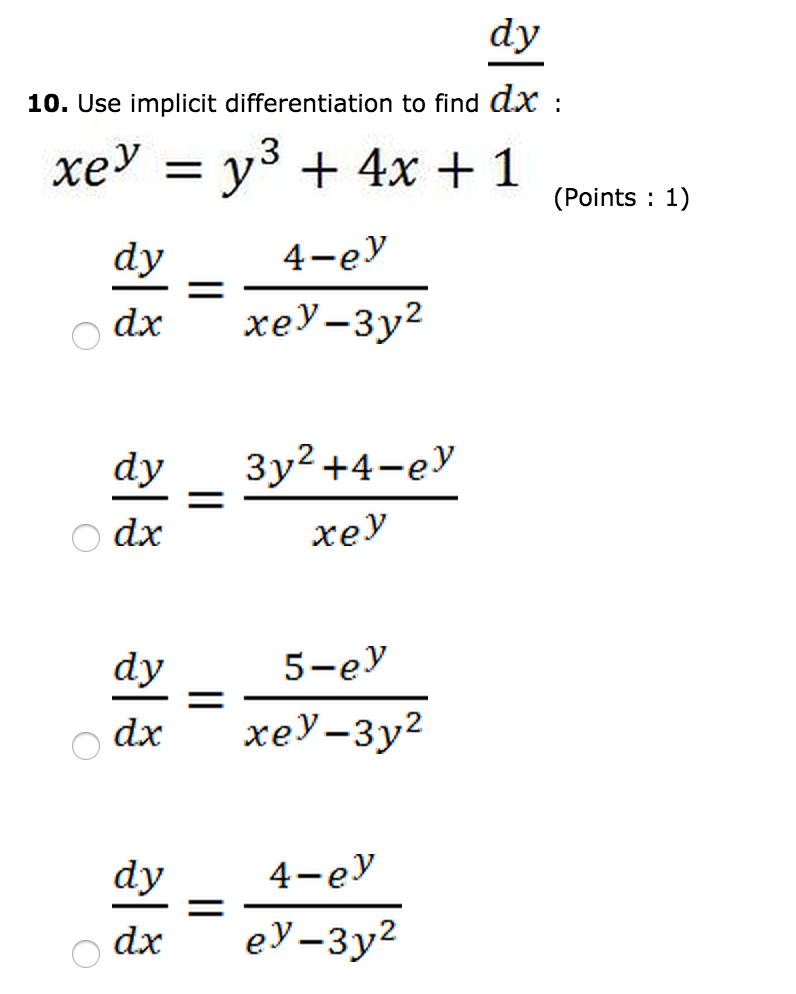
Image: www.chegg.com

Unlocking the Potential: How dx dy Empowers You

Understanding dx dy isn’t just about conquering math exams. It opens up a world of possibilities, empowering you to tackle real-world problems with a newfound clarity.

• Critical Thinking: By understanding how small changes interact, you gain a powerful tool for analyzing complex situations. This critical thinking skill is invaluable in all aspects of life, from personal decision-making to navigating challenging work situations.
• Problem-Solving: The power of calculus to model change and predict outcomes gives you a distinct advantage when tackling problems. This ability to predict and analyze becomes a powerful tool in engineering, finance, and other fields.
• Enhancing Your Worldview: By recognizing the power of infinitesimal change, you develop a deeper appreciation for how the world works. It allows you to see the intricate interplay of forces that shape our reality.

What Is Dx Dy

https://youtube.com/watch?v=-ssDNYmO6DY

The Journey Continues: Exploring Further

This exploration of dx dy is just the beginning. The world of calculus is vast and fascinating, and there’s much more to discover. There are countless resources available to delve deeper into the intricacies of dx dy and explore its applications further. Online tutorials, textbooks, and even engaging documentaries can help you continue your journey into the heart of calculus.

Most importantly, remember that learning is a continuous journey. Don’t be afraid to ask questions, explore different perspectives, and embrace the excitement of discovery. The next time you encounter “dx dy” in a mathematical equation, remember the power it holds and the intricate story it tells. Let it inspire you to delve deeper into the world of calculus and unlock the mysteries of the ever-changing universe.